Lec 20 - Linear Independence & Basis
Friday, February 17, 2012
9:29 AM
![]()
![]()
![]() Linear
Independence
Linear
Independence
Definition
![]()
![]()
![]()
Eg
![]()
Solve the equation
![]()
We get the following homogeneous system of linear equations:
![]()
![]()
![]()
![]()
Row reduce the coefficient matrix:
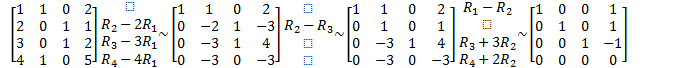
![]()
![]()
![]() Basis
Basis
![]()
![]() What is
the smallest spanning set of a vector space?
What is
the smallest spanning set of a vector space?
![]() Theorem:
Theorem:
![]()
Proof:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() Theorem
Theorem
![]()
Proof:
Prove the contrapositive:
![]()
![]()
![]()
![]()
![]()
![]()
Both theorems together imply the smallest spanning set of any vector space is linearly independent.
Definition:
![]()
Eg
![]()
![]()
Eg
![]()
![]()
![]()
This gives a system of linear equations:
![]()
![]()
![]()
![]()
Row reduce the coefficient matrix:

![]()
![]()
![]()
Since the rank of coefficient matrix must equal to the number of columns, the homogeneous system has a unique solution (the trivial one).
![]()
![]()